How to Find the Diagonal of a Rectangle
Our diagonal of a rectangle calculator is an extensive tool that quickly finds the diagonal and other parameters of a rectangle. Have you met a specific rectangle problem and you don't know how to find the diagonal of a rectangle? Try entering a couple of parameters in the fields beside the text or keep reading to find out what are the possible diagonal of a rectangle formulas.
You can see a typical rectangle in the figure below. We have marked five basic quantities that describe a specific rectangle. You can use them to derive the diagonal of a rectangle formula. These are:
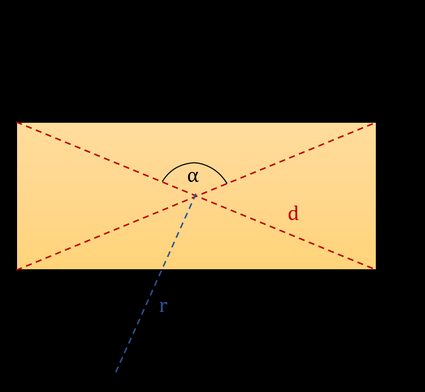
- l - length,
- w - width,
- α - angle between diagonals,
- r - circumcircle radius,
- d - diagonal,
and two other parameters which are not shown in the picture:
- A - area,
- P - perimeter.
The term rectangle comes from the Latin rectangulus that is a combination of two words: rectus (right, proper) and angulus (angle). This name derives from the fact that a rectangle is a quadrilateral with four right angles (4 * 90° = 360°). Its opposite sides are parallel and of equal length, and its two diagonals intersect each other in the middle and are of equal lengths too.
A square is a special case of a rectangle. Its definition is that it has all four sides of equal length, or alternatively, the angle between two diagonals is right. Go ahead on try our calculators dedicated to squares. They can quickly estimate the perimeter, area and diagonal of every square you want, just from the length of its side.
The center of a rectangle is equidistant from its vertices, and therefore you can always circumscribe a circle on it. On the other hand, you can inscribe a circle into a rectangle only if it is a square.
How to find the diagonal of a rectangle?
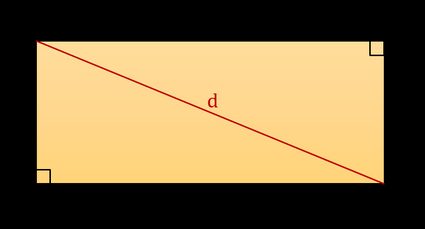
To find the diagonal of a rectangle formula, you can divide a rectangle into two congruent right triangles, i.e., triangles with one angle of 90°. Each triangle will have sides of length l and w and a hypotenuse of length d. You can use the Pythagorean theorem to estimate the diagonal of a rectangle, which can be expressed with the following formula:
d² = l² + w²,
and now you should know how to find the diagonal of a rectangle explicit formula - just take a square root:
d = √(l² + w²).
Our diagonal of a rectangle calculator allows you to use almost any units you want. Check out our length converter or area converter to find out how to switch between different units (SI and imperial).
What's the diagonal of a rectangle formula?
Sometimes you won't have given all of two rectangle's sides. How to find the diagonal of a rectangle in that situation? The answer is provided with our diagonal of a rectangle calculator. At first, let's write down three basic equations, for the area, perimeter and circumcircle radius:
- Area of a rectangle:
A = w * l, - Perimeter of a rectangle
P = 2 * w + 2 * l, - Circumcircle radius of a rectangle
r = d/2.
With the above equations, we can now derive various diagonal of a rectangle formulas that are used by this diagonal of a rectangle calculator:
- Given length and width:
d = √(l² + w²), - Given length/width and area:
d = √(A²/l² + l²)ord = √(A²/w² + w²), - Given lenght/width and perimeter:
d = √(2l² - P*l + P²/4))ord = √(2w² - P*w + P²/4), - Given length/width and angle:
d = w / sin(α/2)ord = l / cos(α/2), - Given area and perimeter:
d = √(P² - 2*A), - Given area and angle:
d = √(2 * A / sin(α)), - Given perimeter and angle:
d = P / (2*sin(α/2) + 2*cos(α/2)), - Given circumcircle radius:
d = 2 * r.
Note: The angle α between diagonals is in the front of the length like in the first figure. Also, remember that the diagonal of a rectangle calculator assumes that the length is longer than the width!
Did you know that there is a special rectangle called the golden rectangle? If not, head on to our golden rectangle calculator and see how you can construct golden rectangles!
How to Find the Diagonal of a Rectangle
Source: https://www.omnicalculator.com/math/diagonal-of-rectangle